Yeesh. Been a while. Back on the first day of school I started taking notes for what was supposed to be my annual “First Day of School” blog post. And then the day just kept getting crazier and crazier. Here’s my day, from a very foggy memory, in neat, numbered-list form:
- Copiers explode (or something like that)
- All-School assembly
- A period (Algebra II)
- B period (Foundations of Computer Science)
- C period… is missing? Apparently, they had locker day the first day (8th grade computers)
- D period… why are the eighth graders all missing? (Algebra I)
- D period… wait, why are the eighth graders at lunch and not in class- oh… oh, dear god no…
- D period… 9th grade goes to lunch and…
- D period… 8th grade shows up. I get no lunch 😦
- E period (Algebra II)
- F period…. PREP! FINALLY! I don’t remember for sure, but I’d bet everything that I got out of the building for lunch, even if I had brought my own.
- G period…. PREP! But not really! We have an away soccer game!
- Soccer away game! We lose! 😥
- I get back to the school around 7pm, home by 7:30pm.
Things of note:
I have five classes, four preps this year. They are the first five periods of the day. Algebra II (twice), Algebra I, Foundations of Computer Science, 8th grade computers. The Algebra 1 class is split; half 8th graders and half 9th graders. This led to the crazy scheduling fluke described above. Basically, at our school D-period is the lunch period which unfortunately and inadvertently was when this class was scheduled. Normally, E period is the lunch period, but on some special days, it is bumped to D period. Basically, I had D period twice and I lost my lunch in the process. Foundations of Computer Science is a new class to our school. It’s AP CS lite for now and it is just a semester long class. The students are learning Java and hopefully the foundational concepts behind all computer programming. 8th grade computers is also a new class to our school. Students are learning the introductory ideas behind programming. We’re “coding” in Scratch. The 8th graders are creating computer games. This is fun. It’s a quarter-long (8 week) class that will get repeated 4 times so that each 8th grader in the school takes it. Our school is one step (AP CS) away from a fully comprehensive, 6-12 computer science curriculum. This is awesome. In a time that most schools have cut CS, ours has added it and gone all-in. This is very awesome.
Coaching a varsity sport during fall semester is tough, even if I’m only an assistant coach. Soccer’s final regular season week is upon us, however it’s likely we’ll go several games deep into the playoffs, so there’s still a few weeks left.
I am loving teaching algebra II. I really have nothing that’s all too amazing to add here. My students are awesome and I’m finally getting the chance to teach a course two years in a row and it is so much fun.
My parent-teachers conferences were da bomb. I couldn’t fit my head through the door after all my students’ parents told me how much of a baller I was. Seriously, though, they went so smooth and mostly because we have awesome kids and awesome parents. I’m very lucky!
The Coolest Thing I’ve Done in the Class So Far
In my 8th grade computer class we took a day, left the classroom, sat in a circle in the hallway and had story time. The book:

The reasoning behind it was an introduction to control statements: if-thens. I cannot emphasize this enough:
Teenagers LOVE reading children’s books!
I think it’s a change to look back at how simple things were for them just a few years ago. They truly enjoy it. I always start by reading If you give a mouse a cookie. I don’t tell them why at first. They love it. They demand I provide ample time to look at the pictures. They sit silently, fully attentive, as if this was the most enjoyable thing they’ve done in a classroom in years (and maybe it is, which says…). I then ask for volunteers to read the other three stories in the series. This takes about 35 minutes total, leaving time to talk about If-then statements.
This works great for geometry classes, but also it worked very well for my 8th grade CPU class. I’ll probably bust it out for my Foundations of Computer Science class as well. I strongly, strongly recommend every math teacher buy the book that has all four stories in it, one of the best purchases I’ve made:
Mouse Cookies and More: A Treasury
It comes with the four original stories and a bunch of songs and recipes that I ignore. I bought it for $25 at a Barnes and Nobel (before educator discount) but you can get it cheap on Amazon at the link above.
Hope everyone’s first month of school has gone well (and maybe a little less busy than mine!)
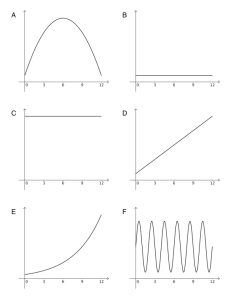 There’s a blog post from Reflections in the Why out there in which the blogger asks “Which graph best represents the importance of teacher knowledge of mathematical content as a function of grade level taught?”
There’s a blog post from Reflections in the Why out there in which the blogger asks “Which graph best represents the importance of teacher knowledge of mathematical content as a function of grade level taught?” 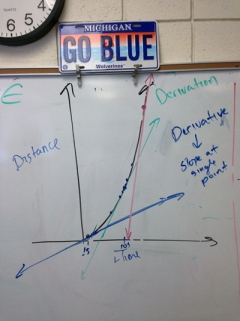 However, it’s still crucial we have that background. Because, then when you’re teaching 8th grade algebra and introduce slope, you can ask the question:
However, it’s still crucial we have that background. Because, then when you’re teaching 8th grade algebra and introduce slope, you can ask the question:
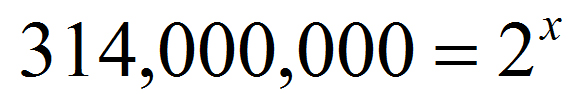










You must be logged in to post a comment.